I admit that I am enjoying teaching undergraduate students. The challenge is enormous. Effectively you're trying to convey big ideas in minimum time to people who have had remarkably little training in the subject about which you're talking.
Take macroeconomics. It's a subject few grasp and which some even deny exists. How do you summarise the key objectives?
First, unless you accept that change in outcomes is possible then there is little point in engaging in the study of macroeconomics, which without that acceptance is reduced to the status of observing phenomena.
Second, you have to ask what the motive for study is. I suggest two things. The first is assisting the meeting of need. That is at the core of all economics. The second is the correction of market failure: that is, putting right what microeconomics gets wrong because of the existence of marketplace failures and externalities.
Third you have to frame these issues into meaningful questions worthy of study. I'd summarise all those question in one phrase: how big should government be to effect the change we need?
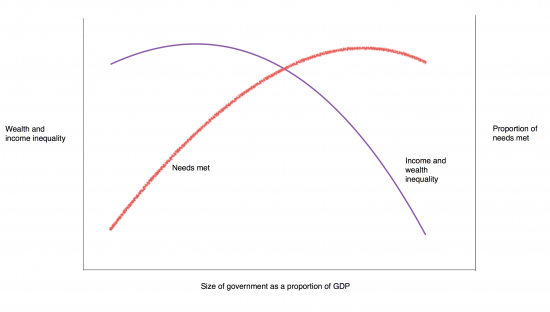
And since economics without a diagram or two is pretty much inconceivable I came up with this:
The left hand axis represents inequality; the right hand the proportion of all needs (not wants) met in society. I stress, needs is not a base line here. It is a measure of being able to take a part in society, but without excess. And the X axis is the proportion of GDP spent by the government.
I have sketched in two functions. I stress, I am being simplistic, not least in assuming a single variable for income and wealth inequality. But they will do for now: the shapes are also likely to be more complex than shown.
It's my suggestion that in the first instance wealth inequality might rise as the proportion of GDP spent by the government rises: the power of the state does, after all, reinforce the ability to enforce property claims. But as the state grows in size so too does its ability to find and tax property on which tax liabilities rightfully arise, and so also its willingness to take on powerful vested interests grow. As a result ineuqality then falls steadily. I could have added an upward kicker at the right hand end of this function: very big government (beyond the ranges seen in most democracies) is often associated with corruption that concentrates wealth.
If is, of course, entirely logical for the state to redistribute in this way: because those on lower levels of income have higher marginal propensities to consume than those on higher levels then income redistribution releases funds into the economy that stimulate growth. Given many governments want to deliver growth (although I think they should be careful as to its composition) then redistribution makes logical macroeconomic sense.
As this process of redistribution stimulates growth then we see the second function, on the proportion of needs met, kick in and rise with the size of government. I stress, this does not mean government meets all such needs but it does provide the environment in which they are met.
Again, in a macro sense this is logical: government spending in an economy broadly does three things. First it does, as a matter of fact, increase GDP unless that economy is operating at full physical and technical capacity. Second, government is the prime mover in a lot of innovation and investment. This kicks into growth via increased consumption and, one hopes, exports. And third, some spending is of course straightforwardly meeting basic need and the security that provides means people need to save less, and so spend more, and so increase economic activity through consumption. In a purely logical sense, all components of GDP are likely to rise as a result of government spending.
So what is the best size of government? Logically it is where the meeting of need is maximised so long as wealth and income inequality is still declining at that point (i.e. the kicker upturn I might have included in the wealth function, noted above has not begun, which would indicate trouble ahead if it had).
It's not more than a doodle, but I would not have thought about it if I had not been teaching. And I think there is some merit in it as it answers a pretty fundamental question.
Thanks for reading this post.
You can share this post on social media of your choice by clicking these icons:
You can subscribe to this blog's daily email here.
And if you would like to support this blog you can, here:



I was told in my undergrad economics degree 30+ years ago in Australia to ignore needs and focus on wants.
Can you please define a need?
Is a need only those things necessary for basic survival (e.g. basic food, water, shelter)? There are tribes in the Amazon and Papua New Guinea who get by on little more than that.
Is it is something more than that? E.g. phones, opera, computer games — which people have lived without for centuries, and still do.
If so, who gets to decide what is a need or a want? You? Me? Politicians? Corporations?
You might like to read The Courageous State
I have not got time to rewrite it here
Sorry
“I was told in my undergrad economics degree 30+ years ago in Australia to ignore needs and focus on wants.”
Sadly that is now the reverse of the problem facing most people in most “developed” countries now!
I would be a very happy semi-retired man if I only needed to pay the same as the tribes you mention for “those things necessary for basic survival (e.g. basic food, water, shelter)”
If as a society we focused on providing needs for everyone at the minimum cost, just think how much we might have to enjoy the wants in this world. Instead most people can now barely pay for their needs and wants are a once a year luxury if that – because our basic needs have been privatised and financialised into modern day luxury priced items!
There is no “optimal” size for a government because this implies a relatively fixed or static situation which is largely predictable and controllable. In our present world this does not happen and we are in a situation of constant change that is complex and only partially predictable. Also, as The Great Duke once said, it is not a case of having more cavalry, it is a case of having better cavalry.
I agree entirely
But I suspect that what I am suggesting would support that view
There is no “optimal” size for a government because this implies a relatively fixed or static situation which is largely predictable and controllable. In our present world this does not happen and we are in a situation of constant change that is complex and only partially predictable. Also, as The Great Duke once said, it is not a case of having more cavalry, it is a case of having better cavalry.
This is a fundamental question and some states may already do what I put forward below.
Here are some principals for a State – things it is there to do.
1. Reducing/negating inequality (you’ve mentioned this of course)
2. Laying down the rules of business, banking etc., and enforcing them (regulation)
3. Balancing the various power interests at play in the economy and polity of the country – everybody should get something but not at the cost to the other.
OK – there could be more principles but 3 is not a bad start. However wrapped into one principle you could say that such a State is aiming to ensure that (say) capitalism is delivering the ‘greatest amount of economic benefit to the greatest amount of people’.
To me the size of the State is determined by how well the private sector is working or not. I wish the neo-libs would realise this. A bigger state actually means a poorly functioning private sector or poorly function capitalism (that which is only creating wealth for a small section of the population).
In my job in social housing, it would not be needed if the private rented and owner occupied sectors were delivering homes to live in at a reasonable prices and the wages paid were adequate to live and save on (amongst other factors.
I would say that in order to fulfil these functions the State needs to be as big as it needs to be at any one time to deal with particular problems.
So if the State were to really tackle the banks and financial sector now, initially it would have to be rather large whilst it brought about a change in ethos and culture; we would certainly not be cutting staff like they do at HMRC.
Then there would have to be some sort of vigilance role which again where it would need to big enough to cope and be effective. Based on the recent really bad behaviour we have seen in the money markets the State would need a big presence here for some time.
So the design size of the state could actually be flexible – it might expand and contract depending on how well the markets were behaving and could shrink when it is not needed – when some sort of optimum state of equality is reached.
Considering recent history though, I think that States will need to grow and be maintained at a larger size for some time. And instead of just bailing out the private sector financially next time (this time?), I would demand that future State intervention brings about the cultural and structural change many of us crave.
Your diagram is almost perfect but perhaps you should have reversed it, as the left side represents right-wing policies and the right side represents left-wing policies. That said, the government agrees with you and is creating policies that bring the greatest inequality and meet the fewest needs. A very important point to raise with your students is that the measure of inequality (Gini), by which relative poverty and childhood poverty are measured, is a complete lie, because it is based on regular income that excludes inheritances received. (They may not be regular but they are certain – we all die). As wealth inequality continues to grow, inheritance will play an ever greater part in income inequality, largely hidden from view.
Agree really Gini
Much prefer the Parma
Did you mean the size of “government” or the size of the state? And is size measured in terms of number of employees, total spending, asset values or something else?
This got me thinking about what economic students are taught about the underlying terminology and concepts of government, state, public sector, private sector, not for profit sector, money, banking, etc etc…. And how these things are measured, what is included, what is not, why not etc etc…
To say economics is complicated is an understatement, and then you’ve got to have a good grasp of politics and social history to make any real sense of it (not to mention maths which complicates the whole thing completely for me).
Good luck to your students, I’m so glad I haven’t got to go through undergraduate life again (apart from the social life of course!)
Proprtionate of GDP
But good points
An interesting approach.
My first reaction was to assert that the important question is: “What economic levers are available to government?”
But, reading the replies, I would say that students – and many who have been taught economics and consider themselves to have studied enough – need to look at simple charts and ask the complex question “To what ends should a government act in the economy?” before they consider how.
We would all do well to consider the results of a ‘captured’ government acting to accelerate the concentration of wealth – knowingly, or willingly in wilful ignorance: the spectre of Mugabe economics is closer than you think.
Government needs enough people and apparatus to govern.
It seems plain to me that issues with tax collection are because HMRC has been cutting staff and closing down local offices.
Housing space standards in new build have dropped because there are less people checking on minimum standards.
The use of derivatives in the United States that helped create the 2008 crash was poorly regulated and regulators were cut back in order to help grow the business.
In this world it seems that too small a Government – especially one committed to equality and looking after the planet – could not do the job.
Government that is too small is a major issue
If too small it has not got the capacity to intervene at macro or micro levels
Some, of course, want that
Government. What is meant in this context?
Or governance? Governance tends to have more of a regulatory nuance.
If “government” is indeed the system by which a state or community is controlled, then surely the minimum is best. Avoids any “crowding out” nonsense.
When it comes to governance, the amount of regulation should be enough to encourage the best outcome for the common good, no?
Sadly the term “government run” seems to have become associated with inefficiency, and “non-government run” with some kind of meerkat’s know best mentality.
I suppose we need some data and definitions.
I suppose we need some reason too, not driven by crazy notions like ‘crowding out’
Provide your evidence please
You say, Richard, you prefer Palma to Gini as a measure of inequality. But I don’t think that takes account of inheritances as a source of income either. The effects of inheritance should not be underestimated; for the recipients it is income, despite which the ONS argue that it is a transfer of capital.
Accepted
But it is better overall
That’s my argument